We’ve been looking at slowing down a starship, pondering ways the interstellar medium itself might be of use, and seeing how the stellar wind produced by the destination star could slow a magsail. A large solar sail could use stellar photons, but the advantage of the magsail is that it’s going to be effective at a greater distance, and we can also consider other trajectory-bending effects like the Lorentz turning studied by Robert Forward and P.C. Norem. But if you take a look at the relevant papers on magsails and other uses of the medium, you’ll find that they all assume the interstellar medium is more or less uniform. We know, of course, that it is not.
For one thing, the Sun itself seems to be near the boundary of the Local Interstellar Cloud, and there are a number of such clouds within about 5 parsecs of the Solar System. In fact, we’re not exactly sure whether the Sun is just outside the LIC or barely within it. In any case, as Ian Crawford has pointed out, Centauri A and B appear to be outside of the LIC in the direction of the G cloud, yet another denser region of the local interstellar medium. Although Robert Bussard assumed densities of about 1 hydrogen atom per cubic centimeter for his ramjet, a starship between denser clouds may encounter far less, perhaps 0.01 hydrogen atoms in the same volume.
The other wildcard is the fact that leaving and approaching a stellar system, we encounter the kind of interesting effects shown in the image below. This is anything but a uniform interstellar background. The bubble created by the solar wind is called the heliosphere, at the outer boundary of which is the heliopause (here the solar wind is balanced by inward pressure from the interstellar medium), and as you can see in the diagram, the bow shock forms on the outer edge as the star moves through the ionized gases of the medium. Still within the heliosphere is the region called the termination shock, where the speed of the solar wind is abruptly reduced — between the termination shock and the heliopause is the area known as the heliosheath.
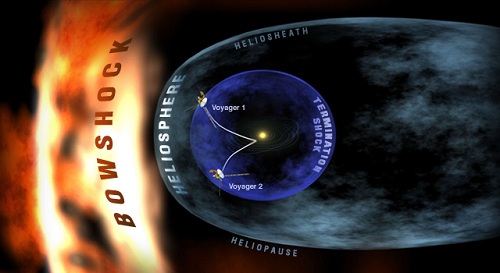
Image: The complicated interactions between the Sun and the local interstellar medium. Credit: NASA/JPL.
Physicist and writer Gregory Benford calls the bow shock, that bumper of plasma and higher density gas that forms 100-200 AU from the star, “the obvious place to decelerate.” Obvious it may be, but I haven’t encountered the idea in the literature before, and it’s an ingenious enough notion that I suspect we’ll be seeing a paper or two on the matter before long. The suddenly higher density and plasma content available here should allow interesting maneuverability along the lines of the Lorentz force turning that Forward and Norem studied for course correction and round-trip missions. The bow shock should also offer prime ground for deceleration.
We have early data on the termination shock from Voyager 2, which crossed it at 84 AU back in 2007, while Voyager 1 entered the heliosheath at 94 AU in 2004, and Benford figures the plasma density increase at the bow shock should be one to two orders of magnitude above the interstellar density, and that means one or two orders of magnitude more deceleration. I want to quote him on this from a recent email:
My main point is that these are 3D structures, so a starship could navigate through them using the Forward I x B torque model which steers without decelerating. Each of the bow shock, heliopause and termination shocks are surfaces one can sail on and in, maximizing the deceleration.
So here is the method for the star sailors of the far future:
I imagine that any trial of a starship in, say 100 years, will begin with expeditions into the several hundred AU shock environment, have a look at distant iceteroids and maybe dwarf stars. Then turn back and try to decelerate using magsail skills on the shock surfaces available. (I surf, and this is like inverse surfing, using natural wave phenomena to slow.) Develop the tech and skills to sail the interstellar seas!
As a starship approaches a star, sensing the shock structures will be like having a good eye for the tides, currents and reefs of a harbor.

Image: Spitzer image and artists conception of the bow shock around R Hya. Credit: NASA/JPL, Toshiya Ueta.
Now we can look at certain astronomical images in a new light, as witness the Spitzer imagery and subsequent artist’s concept above. This is the star R. Hydrae in infrared, showing the bow shock about as well defined as I have ever seen it. Approaching a star using these decelereation methods would involve a long period of braking moving through and along the bow shock, heliopause and termination shock, staying within the high density plasma to take advantage of the increased densities there with the starship’s magsail fully deployed. After the long spiral into the inner system, continued magsail braking or perhaps inner system braking using a solar sail would allow the vehicle to maneuver and explore the new solar system.



This is probably a stupid question, but what if the bow shock is on the other side of the star system from your ship? Or is that were stuff like Lorentz force turning comes in?
Interesting idea. Given estimates of the bowshock’s size and density, I suppose that we can estimate how fast a ship can enter it and still expect to slow down and orbit the star, rather than just skipping off back into interstellar space. Does Dr. Benford have any preliminary estimates of the order of c velocity that can be braked in this way – 0.001c, 0,01c, 0.1c, higher?
R Hydrae has an asymmetric bowshock? How does that work?
I really appreciate the series of articles this week, Paul. I’ve been exasperated at the flyby-only interstellar mission concepts I’ve read about. Why spend almost countless amounts of money and time traveling to a stellar system just to pass right through it in a relative eyeblink? The public would only support and get excited about a mission that can decelerate a probe, robotic or human-carrying, that can stay in the target system and explore for a while. The articles this week gives me hope that a long-exploring probe (think at least Cassini) would be possible.
As a science fiction writer, I believe it’s important to understand the “geography” of the Solar System. These last few posts have really helped me do that. Thank you.
Mike and James, thanks for your comments — glad these articles are proving useful!
Alex: Re how much braking can be applied, I think we’re too early in the game to know. Someone needs to go ahead and run the relevant calculations in different environments. Benford has recommended working the numbers for our own magnetosphere bow shock toward the Sun, Jupiter’s bow shock and, third, the termination and other shocks at ~100 AU to ~200 AU from our star. But to my knowledge no one has run these calculations yet re braking and maneuverability.
Curt Wohleber writes:
Good point, Curt. You can see that the star’s relative motion becomes an important factor in this calculation. But Lorentz turning does at least offer the option of changing a trajectory depending on how the star is approached, and certainly abundant ways of maneuvering once the vehicle is within the shock area.
How flimsy are these deceleration devises? To my mind anything this size must be very robust to withstand 1m/s/s, and this would require 3000AU to halt a probe that was travelling 10% c, which is an order of magnitude higher than the scale given here. Actually, just the centrifugal forces from trying to turn around without slowing at this distance would be 60m/s/s for 0.1c, thus many times higher than Earths gravity.
@ Rob
I calculate a turn of 100AU radius at 0.1c at ~ 60m/s/s is ~ 6g. 600 AU would reduce that to 1g. The duration of the turnaround would be ~ 100 days at 600 AU.
As you point out, this is some serious stress on a spacecraft. OTOH, I like the idea that it might be possible to decelerate at 1g. (Now if only we could accelerate at that rate too).
@Paul. I would assume that it is possible to compute forces as a function of medium density. This would at least indicate the magnitude of the forces we might expect given certain conditions.
How flimsy?
Good question Rob, my understanding is that the mag sail conductors only have strength in tension (maybe a lot of tensile strength if they are made of doped carbon nanotubes?) and it is the field force which is supposed to keep the sail from collapsing like an umbrella in a gale, but I have not run the numbers.
Hi Rob
Mag-sails have to be strong to withstand the hoop-stresses caused by their magnetic fields and can be engineered strong enough for a few gees if need be. They don’t necessarily mass very much for their immense size, so the stresses on the tethers attaching them to a space-vehicle don’t need to be excessive either.
Incredibly , the more we learn about it , the more strange becomes the parallel between our hypotetical interstellar sailing ship and the wooden sailingships of past glory . In order to sail long distances the crew was mostly bussy changing the configuration of the sails ,maintenance , dealing with friction issues on the hull , and sometimes using completely diferent tecniques such as driftankers , using oil to to change the viscosity of water ,and deploying extra “finns” to reduce drift . And , ofcourse , they were stearing by the stars too …
Our starship will need a very active crew as well ,if it must be capable of changing between several propulsion -modes a number of times , and perhabs remanufactoring the next propulsion system from the materials of the former .
Rob and Alex: You are making a good start on getting actual numbers. However, I think accelerations in the range you have in mind are out of the question. Remember that any acceleration must be gained by pushing against something, and there just isn’t much there, bow shock or not. They don’t call it empty space for nothing.
Dana and Zubrin estimate in their 1990 paper that up to 0.01 m/s^2 can be achieved with a magnetic sail in solar wind at 1AU. Plug that into your work, and you get one healthy dose of reality check. Now think that at 100s of AU the plasma density is much, much less than at 1 AU, and that Dana and Zubrin did not take into account the collapse of field lines under force, and you should realize that this is not the panacea some are hoping for, not by a long shot.
Similar caveats apply to Lorentz turning. The fields are really weak, and so are achievable charge/mass ratios. There might be some promise here, but perhaps only in the direct vicinity of stars and planets with a magnetic field.
My guess is that the reason you see so little quantitative work published on this is that the ideas prove unworkable after a back-of-the-envelope treatment, and backs of envelopes are hard to publish.
Here is what my back of the envelope says about Lorentz turning:
The idea as I have heard it is a charged wire, with the charge created and maintained by a beta emitter. Beta radiation gets up to about 1 MeV, which limits the voltage you can have on the wire until the electrons just turn around and come back to 10^6 V. The capacitance of a 1mm wire in the interstellar medium assuming a Debye length of around 7 m (which I found here: http://www-astro.physik.tu-berlin.de/~breitschwerdt/teach/ism_03_db.pdf) is roughly around 10^-12 F/m. The mass of same wire at water density would be around 10^-3 kg/m. Charge is voltage times capacitance, so we get a charge/mass ratio of 10^-3 C/kg.
Now consider the Gyration frequency: The frequency at which a charge circles in a given field. Its inverse is the time it takes to complete one full turn. It is given by the product of magnetic field strength and charge/mass ratio: W = B * (q/m). With a typical galactic magnetic field strength of 1 nT, we obtain a gyration frequency of 10^-12 seconds, or one complete turn in 30,000 years.
There is some room for improvement, especially making the wire thinner while keeping enough strength to hold the force could gain us some orders of magnitude in q/m, so this is certainly not the last word, but it is still not very encouraging.
That should be 10^-12 per second for the gyration frequency
The above is a little unsatisfactory, because it apparently lets us increase the q/m ratio indefinitely by simply making the wire thinner and thinner. There must be a limit to that. Perhaps material strength? Here is another back-of-the-envelope:
The energy in a capacitor is 1/2 C V^2 or 1/2 Q^2/C. As before, C = e*l, where e = 2 pi eps0 / ln (a2/a1). This varies weakly with wire radius, but we’ll assume it constant for now, as before at e ~ 10^-12. The strain on the wire will be the derivative of the energy by length, i.e. F = (1/e) Q^2/L^2 = 1/e q^2, where q is the linear charge density in C/m. This force can not exceed the yield strength of the wire Fy, which is given by the specific strength S, density rho and cross section a as Fy = S*rho*a. Here, S has units (N/m^2)/(kg/m^3) which works out to m^2/s^2, or velocity squared. The maximum specific strength of known materials goes up to a few million, let’s say 5*10^6 m^2/s^2.
Linear mass density: mu = rho*a kg/m
strain: F = 1/e (q/mu)^2 * rho^2 * a^2 <= S rho a
This works out to a maximum charge/mass ratio of
(q/mu)^2 = e S / mu ~ 5*10^-6 / mu
The units also work out:
[C^2 / kg^2] = [C^2 s^2 / m^3 kg ] [m^2 / s^2] / [kg / m]
Interestingly, we still have the situation where we can make the wire thin and gain q/m, but now it is only a linear relationship, before considering strain it was quadratic.
What does that mean in terms of time to complete a full turn? With a 10 micrometer wire, we would get a linear mass density of ~10^-7 kg/m, which would result in an (incredible ?) q/mu of seven Coulomb/kg. This would shave off almost 4 orders of magnitude from our previous result, resulting in a circle-time of merely around 5 years. I am beginning to be excited by this….
As an additional benefit, if the wires were arranged in the shape of a net, the arrangement could double as an electrostatic sail, as described here: http://www.space.fmi.fi/~pjanhune/Esail/paper1.pdf, albeit with a much higher charge necessary to affect Lorentz turning.
As an additional explanation: I have used the circle-time as the inverse of the gyration frequency because it is an expression of Lorentz turning that is independent of velocity and easy to understand. At 0.1c, a five-year circle would be 0.5 light years in circumference, which means you could easily maneuver from star to star, or fly a round trip without extra expenditure of propellant. There will be some energy needed to keep the wires charged, this could be a problem depending on the density of the ISM. The less dense, the better, ironically. Ideally, integrating a high energy beta emitter into the wires will do the trick simply and efficiently.
There is also potential for acceleration. Magnetic fields of planets are much stronger than those pervading interstellar space (here assumed to be 1 nT). That would seem to open the possibility of forced orbits around such planets. For example, the magnetic field near Jupiter appears to be around 10^-3 T, 6 orders of magnitude higher than interstellar fields. The same craft, then, could use Lorentz force to cycle around Jupiter in a few minutes, which makes for velocities of 1000’s of km/s that could be built up slowly using solar system resources external to the craft, rather than propellant. Solar wind, for example, using the electrostatic sail. Or from Jupiter’s magnetic fields, electrodynamically. I know we have mentioned this idea here before, a long while back, but I can’t remember when or how.
With the Lorentz force coming out so unexpectedly favorable, I decided to take another look at magsail deceleration. I looked up some parameters for a magsail here: http://casa.colorado.edu/~danforth/science/magsail/magsail.html
sail radius = 10 km
wire radius = 6 mm
material Ba2Cu3O7Y
max. current= 10^6 Amps
crit. temp = 90 Kelvin
center field= 6.28×10^-5 Tesla
sail mass = 36,000 kg
Where several different configurations are discussed:
Config. I(amps) deltaV/#part. #part. Xsection(m2) Fractional Xsection
axial 50k .031 250 2.8e5 0.001
axial 1M 2.48e4 200 4.97e8 1.58
normal 50k 1.63e4 200 3.2e8 0.76
normal 1M 4.7e5 200 3.9e10 30
I also assume the density of the ISM from here: http://arxiv.org/pdf/1010.4823.pdf as D = 0.2 cm^-3
Then an upper limit of the deceleration force is easily calculated as the momentum per time of the part of the ISM caught in the best case cross-section:
F = a * v * D * mp * v = 4 * 10^10 * 3* 10^7 * 0.2 * 1.6 * 10^-27 * 3 * 10^7
~ 1.2 * 10^4 N
Where:
a: cross section of sail, 10^10 m^2 from above
v: velocity of sail 0.1 c or 3*10^7 m/s
D: particle density of ISM = 2 * 10^5 m^-3
mp: proton mass = 1.6 * 10^-27 kg
We get a force of 12 kN on 36,000 kg, which amounts to an acceleration of 0.3 m/s^2. If this acceleration did not decrease with decreasing velocity, it would take 10^8 seconds, or about 3 years to get to a stop. It does, of course, so the time would be much longer, and there will be the dreaded “doldrums”.
So, it looks like there is actually hope here, although I have used some rather generous assumptions. Particularly, the sail cross-section seems large for a 10 km loop. More detailed calculations are definitely in order. Have those been done? I could not find any on-line…
If such techniques can be used for turning a few degrees at even higher speeds, it sounds like the idea of a cycler moving passengers in comfort around a ring of inhabited worlds is also viable for the far future.
Rob, if there is any truth to these calculations, it would be easy to have cyclers go in arbitrary “schedules” from star to star, like buses go from square to square. But is this useful? To get on you have to accelerate to cruise velocity, to get off you have to decelerate. Each will take years and cost oodles of energy. The cycler does nothing to solve this main problem with interstellar travel. Even if it has a fuel depot. The fuel, after all, must still be brought on, or not?
Re magsails, Eniac writes:
I’m hoping Greg Matloff has some updates on this — we’re due for another interview with him, which I plan to set up soon to discuss magsails, among other things.
Eniac, you missed the point of a cycler. These were never designed to solve the problem of acceleration and deceleration per se, but to show that if we can ever accelerate the passengers to this speed, they can then travel in comfort, as if on a cruse ship, rather than in the cattle-class transporters that economics would otherwise dictate they must settle for.
Rob, most interstellar mission profiles call for years of acceleration and deceleration, so the “comfort” would be limited to a comparatively short time in between. This also means that most of the time, the cycler would be empty or occupied only by “staff”, who are not going anywhere. Any supplies necessary to sustain the comfort would also have to be accelerated separately, so the economics would not be all that different and still dictate cattle-class.
Eniac, those mission profiles are constrained by the original conditions from which they are designed to work. My expectation is that, given an incentive to accelerate a package of minimal mass that can just support passengers through the acceleration phase, the length of this phase will be much shorter, though its final velocity the same. If not all is lost.
Your point about the added payload still having to provision each set of passengers over the entire journey sounds a real killer, until you realise that, in this new setup, this only entails providing enough uranium to power the elaborate food production, and other life-support modules already in place.
Rob, now you are suggesting an entire economy accelerated to relativistic velocity, which requires all of its raw materials to also be accelerated, continuously. Not just the uranium, but also the steel and concrete for building and maintaining the reactors, the dirt and/or water needed to grow the food, etc. etc. All this just to make part of the journey (the cruise part) more comfortable for travelers.
Note that with passengers, the length of the acceleration and deceleration phases is going to be years no matter what, because it could not be done at substantially more than 1 g, and at 1 g a year is about what it takes.
Yes Eniac, I am saying an entire economy accelerated to relativistic speeds. Note that we have previously talked of the potentially large size of an industrial seed, but I believe that this is not the case here since we are free to add any light replacement parts at each pickup. Manufacturing needs are thus very much lower.
I am also surprised that you invoke that a (similarly?) high bulk of infrastructure would be needed for a nuclear reactor when mass minimisation becomes the new design requirement. Was that just an off-the-cuff remark?
Humans should be able to cope with 2g, but if we are immersed in water (perhaps in strong light weight suits or coffins) I imagine that 10g should be fairly easy to withstand. My guess is that if we make them breath liquids we could get to 20g before the density difference between bone and flesh was worthwhile addressing.
Finally, I would like to also note that some destinations may align such that some passengers travel more than one section on that part of the route. Actually passengers here have the option of changing their minds on which stop they will get off on after a few months of travel.
I should have addressed the minimum biomass requirements per person in a self replicating system. The most efficient ecosystems that I are in the marine environment where each step up the food chain typically means a tenfold reduction in biomass. Off the top of my head, I remember the incredible claim that single celled plants in some of these can support a greater biomass of herbivores, than of themselves. Homeotherms put a greater strain on the system than this, but I can still see half a ton per passenger being sufficient to supply all biomass necessary for a complete ecosystem. Just to be sure, you might add another half ton of composting material to that, but I think that unnecessary.
The crucial difference here is that the industrial seed is supposed to fall on fertile ground, i.e. some asteroid or planet that has all the raw materials needed for it to grow. Here, where you are going is relativistic speed, and there is no raw material there. Zero, zilch, nothing. All has to be brought, at extreme cost.
Even at 20 g you would still need many weeks of this. Not my idea of comfortable travel.